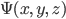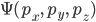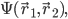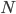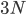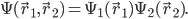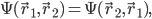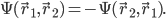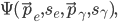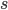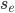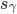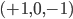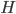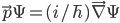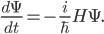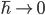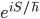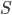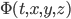Introductory Warning: please note that when theologians call God "simple" that's a technical term. It doesn't mean he is easy to understand. It means that he is not composed of any parts of any kind. (Depending on who you talk to, this could also be taken to imply some stronger statements which are believed to follow from that, e.g. that all of God's attributes are really different ways of talking about the exact some thing, and that they only appear different to us from our limited earthly perspective.)
A certain St. Matthew J. Thériault, whom I met at Ratio Christi, sent me the following questions about whether divine simplicity is compatible with other Christian doctrines such as God's Omniscience, the Trinity, and the Incarnation:
Attached [click on this for a word file---AW] is the abstract to a presentation I intend to deliver at Ratio regarding the doctrine of Divine Simplicity. Regarding the Trinitarian objection there's no relation to physics, and you already personally addressed the Incarnation objection when you last visited Ratio (though I'd be interested if you've given any more thought to the matter of the simultaneity of the ascended Christ and the Church on earth). However, I imagine you'd be able to offer immense insight regarding my objection to Omniscience, which is partially informed by a short article linked within. Thank you in advance for any feedback you might be able to offer.
My reply email spiraled out of control, and having gotten permission to share it on my blog, I will now do so:
Dear Matthew,
Although as you say the simplicity of God is easier supported by philosophical than by scriptural arguments, nevertheless there is a little bit of Scriptural support for the doctrine of simplicity. Traditionally, the doctrine was held to be taught in the Shema: "Hear O Israel, YHWH your God is one YHWH" (Deut 6:4). Jews interpreted this to mean not just that there are no other gods, but also that this God has some kind of absolute unity of being. Traditional Christianity, rather than deny this interpretation to make room for the Trinity, has tended to affirm it and then to assert that the Trinity does not contradict it, because the sense in which God is three is different from the sense in which God is one (the persons of the Trinity are not parts, or additional deities).
One could also gather some indirect support for divine simplicity from the tendency of Scripture to sometimes refer to God as "I am [attribute]" or "God is [attribute]". Also, obviously a lot of the philosophical arguments depend on God's self-existence which is taught more explicitly by "I am who I am" in Ex 3, John 8, among other passages.
Nevertheless, I think one needs to proceed cautiously. While it would be heretical and unreasonable to say that God is actually divided into parts, it seems to me that some theologians have subscribed to extensions of the doctrine of simplicity which go beyond what can necessarily be deduced from it. As you know the very strongest formulations of divine simplicity can lead to a number of philosophical paradoxes which are difficult to resolve.
We must always remember that (whether we are talking about Natural Theology or Scripture), "for now we see through a glass, darkly; but then face to face: now I know in part; but then shall I know even as also I am known", and also that "no man shall see my face and live". Mortal human beings are not capable of seeing the divine nature directly, but only deducing its existence through either revelation or more remote lines. God may be known to exist from philosophy but he is also the Invisible and Incomprehensible Glory, the numinous Sanctus, that haunts all our experiences but can never quite be contained in any of them.
Therefore we should not be surprised if different attributes of God seem to be in some degree of tension with each other; in fact it would be more surprising if we could fully understand how all of the various attributes can be consistently combined. Christianity has never shied away from paradox, as can be seen from the doctrines of the Trinity and the Incarnation. A paradox is not a logical contradiction per se, it is only a sign that our own understanding is limited, yet we can make progress by approaching the same thing from two or more viewpoints which appear to give conflicting information (just like our two eyes, by seeing slightly differing data, can produce a 3d stereographic perspective).
As St. Lewis said about a different theological quandary: "Heaven will solve our problems, but not, I think, by showing us subtle reconciliations between all our apparently contradictory notions. The notions will all be knocked from under our feet. We shall see that there never really was any problem."
What I have just said about paradox is really my primary answer to all of your questions. It would be grossly misleading if I gave the impression that I could 'resolve' paradoxes in the divine nature in the sense of providing a clear logical schema in which the nature of divinity could be fully grasped with the mind; any such scheme would necessarily be misleading and even impious. But nevertheless, as a secondary matter, I think I can say some specific things to resolve some of the specific difficulties you list:
A. Omniscience. This is only an issue if you assume that God's knowledge is, like ours, representational, that is, that it proceeds by means of making something like an image or duplicate of the object known, in some other physical system (in our case, the brain). That viewpoint seems excessively anthropomorphic, and I have already argued against it here:
Fundamental Reality VII: Does God Need a Brain?
To put it into bite sized arguments, God's knowledge cannot be representational because:
1. The Redundancy Objection: If God is omniscient then his thoughts about the universe would necessarily be an exact copy of the universe not differing in any details. But that is silly because if two things are identical in every respect, they may as well be identified. Furthermore it would imply that God's knowledge is limited to the form of the thing (the structural attributes which are the same between the image and the reality) rather than the essence of the thing (which would not be shared between the universe and the image in its mind). But that would be a limitation on God's intellect. So instead we must assert that there is no division between God's knowledge of a thing, and the thing itself.
2. Infinite Regress Objection: Even human knowledge is not purely representational, because that would threaten an infinite regress. For example, suppose we look at a lamp and form a mental image of the lamp somewhere in our mind/brain. We are then aware of the lamp outside ourselves by means of the lamp inside. But how is it then that we know the lamp inside? By means of a second image of the lamp inside of us? That would threaten an infinite regress. Instead we must somehow have the power to directly perceive, without any intermediary representations, some things that reside in our own brains. But God would have the power to directly perceive anything, without any limitations.
3. And of course because it contradicts Simplicity, as you point out. But that just means we have the wrong model of how God's knowledge should work.
I think the article you link to ["Information Storage and the Omniscience of God", by Hollis R. Johnson & David H. Bailey] is completely off-base when it proposes that God's knowledge must be understood as if God were a giant computer. They should have realized that it was this ridiculous idea of their own which they were refuting, and not anything in the Bible or theology as traditionally understood. They say that:
"Some defenders of the traditional doctrine of God’s omniscience may respond to this argument by simply declaring that God is omnipotent and thus omniscient, in the sense of residing and operating completely outside the confines of the Universe and the natural laws that govern our Universe. In short, they may assert omnipotence and omniscience by fiat: God can store knowledge, even an infinite amount of knowledge, without any plausible physical storage mechanism or medium. This is because God’s ways are not ours, and our finite mortal minds cannot possibly hope to comprehend the means employed by this supreme Being. Against such reasoning there is no counter argument."
The reason there is no counterargument against this position is that it is obviously correct. The idea that God is outside the confines of the physical universe and therefore does not store his knowledge on some physical medium such as a film reel, is not some arbitrary stipulation made to avoid falsifying Theism. It is part of the definition of Classical Theism that God is outside the universe, for goodness sakes. To explain God's knowledge by means of ordinary causal mechanisms, far from being a "scientifically tenable theology" would simply be the denial of classical theology, which holds that God as creator is not subject to the limitations and natural laws which govern creation. If we found the giant film reel it would refute, not confirm, Classical Theism. One may as well say that any theism compatible with modern biology would need an evolutionary explanation of why God the Father has a beard!
[Not in my original email: I looked it up, and the authors are actually Mormons. That explains a lot. Mormons are polytheists who believe that God the Father is merely an exalted human being, one of many deities who worked his way up in some sort of cosmic pyramid scheme, and that he has a physical body. So according to them God isn't omniscient and probably does have a literal beard, and a brain with finite information storage capacity. This is, of course, completely different from the classical Jewish-Christian-Islamic concept of God as the absolutely powerful and wise being who is the source of all existence. Such a being, if he happened to exist, would not be a God at all in the traditional Classical Theist sense. Why should I worship a being simply because he happens to be a finite amount more powerful, wiser, or moral than I am? There are already human beings who are better than I am, that doesn't mean they are worthy of my worship!]
B. Trinity. Here I think I need to quibble with some of your language.
While it is true that the Second and Third Persons in some way originate from the First, I do not think it is orthodox to say that only the Father is the First Cause, apart from the other two persons. That would seem to gloss over the crucial distinction between "making" and "begetting". The Father is not a separate metaphysical entity from the Son and the Spirit, indeed the Father has no separate existence apart from his act of begetting the Son and breathing forth the Spirit, since these acts were by metaphysical necessity; it could not have been otherwise. (This does not mean it was involuntary, for God is spirit and his acts are therefore by will and love, not physical compulsion.) The persons are so united that you can't have any of them without having the other two as well. For this reason, I would say that the Triune God taken as a whole is the First Cause, rather than the Father alone.
John 5:26 says that "For as the Father has life in himself, so he has granted the Son also to have life in himself", that is even though it is a gift, the nature of the gift is that the Son has life intrinsically, according to his identical divine nature, rather than derivatively and externally through grace. Otherwise Christ could not say, in the divine sense, "I am", but rather should have said "I was made to be".
As you recognize the persons of the Trinity are not parts (since they are indivisible), but they are real distinctions in the divine nature. It therefore seems inevitable that the analysis of the "relations" which define the persons (things like Paternal, Begotten, and Proceeding) must necessarily differ from the divine "attributes" such as power, wisdom, or love which are common to all persons because they belong to the single divine nature. To that extent I agree with you.
But if this admission seems to contradict some specific analytic formulation of "Property Simplicity", why not simply acknowledge an accidental misstep, coming from an over-strong formulation of Simplicity, and retreat to a slightly weaker version of the doctrine? For example, one might tentatively say that if God is One, then any of his properties must either be identical to himself OR ELSE to one of the persons, and then say that the latter possibility does not contradict simplicity because each person of the Trinity contains the other two within by "perichoresis".
You say that "Begotten" is "unidentifiable with and alien to" the First Person, but this seems to be stating it too strongly, since even though the Father is not Begotten he does have the reciprocal property of "Begetting", which is the exact same thing viewed from the other side. The one implies the other. Apparently God can have real distinctions within himself, but only involving relational terms, of the kind we are discussing.
Thus, since the doctrine of the Trinity is clearer in Scripture than the doctrine of Simplicity, we should adjust the latter to make room for the former, but without of course abandoning the doctrine of Simplicity altogether! Implicit in this is the idea I sketched in my introduction, that God is mysterious, and that the philosophical "proofs" of his attributes, while perhaps compelling, do not amount to strict logical implications. And therefore that there is room for "adjustments" in our very provisional understandings when we run into trouble!
C. Incarnation. As you note, I was asked about this during the Ratio Christi meeting, but for clarity I'll repeat myself a little. According to the Chalcedonian understanding, the Incarnation involves the union of a complete divine nature with a complete human nature into a single person, Christ. Properties like Simplicity would apply only to the divine nature, and therefore it does not contradict Simplicity to note that Christ's physical body had parts and could change etc.
After the Resurrection and Ascension, Christ continues to have a human nature, but now his body and soul are glorified, possessing additional abilities and attributes. This glorified Resurrection body transcends our current earthly state (although we too will be glorified when our bodies are raised from the dead.) I would love to know more about this but our data from the Gospels and Acts is limited. What we do know is that Christ's body was capable of being recognized (though not always immediately) and touched, that he could speak and eat, and that he was capable of teleporting instantaneously. After which he ascended into "heaven" (i.e. somewhere else outside of spacetime as we know it, in which God's will is more fully done as in the Lord's Prayer, as in the angelic world), which he conceptualized as a return to the Father from whom he came, triggering the pouring out of the Holy Spirit on the Church.
Obviously this cannot be understood in an excessively anthropomorphic way. While Christ has a body by virtue of the Incarnation, God the Father does not, and therefore "sitting at the right hand of the Father" cannot be taken literally, to mean the proximity of two physical bodies at a common time. Instead it is an Aramaic way of saying that Christ is placed in a position of full welcome and authority, that a formerly crucified and rejected man is now being given the governance of all Creation, with rebel angels and authorities now fully subject to him. (Try searching the Psalms for "right hand".) Christ's body continues to have an objective and real existence—and our earthly imaginations cannot conceptualize this except by imagining him residing in something like a "place"—but the nature of that "place" is not one that we can understand, until we ourselves follow him there (John 14:2-3). There is no reason to think that the visible universe as we know it is anything other than a small portion of God's creation:
"There may be Natures piled upon Natures, each supernatural to the one beneath it, before we come to the abyss of pure spirit; and to be in that abyss, at the right hand of the Father, may not mean being absent from any of these Natures – may mean a yet more dynamic presence on all levels."--St. Lewis, "Miracles" (essay in God in the Dock).
How "time" works in this "place" is not something which I think we are in a good position to know. I agree that Einstein's theories suggest that time is a feature of our own material universe, so that a completely disconnected universe would probably have a different timestream, if any. However, if there are interactions between two such universes, then there would presumably still be causal relations between them, and hence (I suppose), some partial notion of prior/posterior events.
This agnosticism about the details might seem a little limiting, but I am afraid it may be the best we can do right now, fun as it may be to speculate on the details of the "control room" from which Christ currently reigns!
I am not sure why you think that there needs to be any "simultaneity" between Christ's body in heaven and the "physical universe in between his Ascension and Return", any more than there needs to be simultaneity between the eternal God and us, in order for God to answer our prayers. Christ is present in the Church in a number of ways; as the Head who gives the Body life, through the presence of the Spirit, sacramentally in the Eucharist, and so on; but none of these ways seem to involve or require any one-to-one map between individual moments of Christ's existence in heaven and our individual existence on earth. Even Christ's everlasting intercession for us comes not through continual labor, "offer[ing] sacrifices day after day", but rather by presenting his wounds to the Father once for all, as an eternal atonement for the sins of the whole world: past, present, and future. We may be sure that he knows and cares for all of our needs, which suffices for practical spirituality, without getting into the mechanics of exactly how his glorified human nature shares in the universal knowledge of his divine nature. Psalm 131.
All right; this has become quite a treatise. Hope it helps!
Blessings,
Aron
PS Do you mind if I post this exchange on my blog? This could involve as much or little of your identifying information and words as you wanted.